While perusing geometry textbooks, I would often get bogged down by the intricate diagrams and abstract concepts. But one particular section on the relationship between three straight lines – ab, cd, and ef – caught my fancy. It sparked within me a curiosity that led to a deep dive into the fascinating world of geometry.
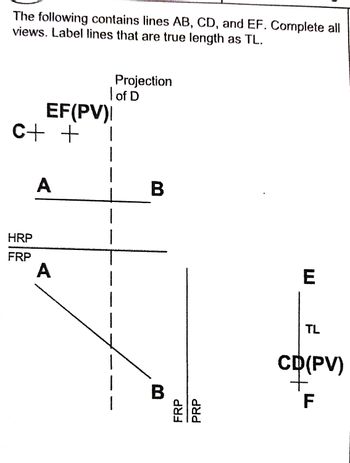
Image: www.bartleby.com
A Geometric Dance: Intersection, Parallelism, and Perpendicularity
The relationship between ab, cd, and ef is a captivating exploration of the various ways in which these lines can interact. These lines can intersect at points, run parallel to each other, or intersect at right angles, known as perpendicularity.
Intersecting Lines: A Tale of Common Crossing
When two or more lines intersect, they create a common point. In the case of ab, cd, and ef, the lines can intersect at as many as three distinct points. These points of intersection divide the lines into segments, creating a multitude of shapes and angles.
Parallel Lines: Never Crossing Paths
In the realm of geometry, parallel lines are fascinating entities. They are lines that lie in the same plane and never intersect, no matter how far they are extended. When ab, cd, and ef are parallel, they coexist harmoniously, running alongside each other indefinitely without ever meeting.
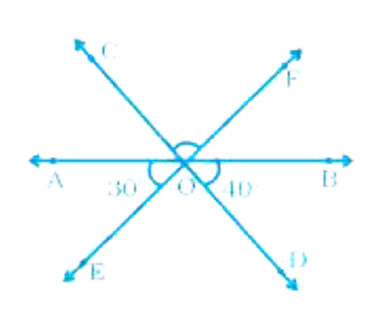
Image: www.doubtnut.com
Perpendicular Lines: An Interplay of Orthogonality
When two lines intersect at right angles, we encounter the concept of perpendicularity. In the case of ab, cd, and ef, if two of the lines are mutually perpendicular, they form a right triangle and exhibit a unique relationship of orthogonality.
Exploring Real-World Applications: A Tangent Diversion
The relationship between ab, cd, and ef extends beyond the confines of geometric diagrams. It finds profound applications in various fields, including architecture and engineering. Tangent lines, a notable offshoot in this geometric exploration, play a crucial role in calculus and various aspects of modern mathematics.
Delving into Parallelisms: Modern Implications and Geometric Art
The notion of parallel lines has captivated artists and architects for centuries. From the iconic pyramids of Egypt to the sleek skyscrapers of modern metropolises, the use of parallel lines creates visual harmony and spatial balance. Parallel lines are not only visually appealing but also a testament to the mathematical precision that underpins architectural masterpieces.
Expert Insights and Practical Tips for Geometric Exploration
As a seasoned blogger and geometry enthusiast, here are a few tips and expert advice I have accumulated over the years:
- Visualize the concepts using diagrams and sketches.
- Use colors to differentiate and emphasize different lines.
- Simplify complex shapes into smaller, manageable components.
- Break down problems into smaller steps to enhance clarity.
By incorporating these tips, you can transform your geometric exploration into an engaging and enlightening endeavor.
Frequently Asked Questions (FAQs): A Comprehensive Glossary
Q: What is the necessary condition for two lines to be parallel?
A: Two lines are parallel if they never intersect, no matter how far they are extended.
Q: When do two lines intersect?
A: Two lines intersect when they share a common point.
Q: What is the relationship between perpendicular and tangent lines?
A: Perpendicular lines intersect at right angles, while tangent lines intersect at only one point, without crossing each other.
Ab Cd And Ef Are Straight Lines
Conclusion: Embracing the Geometric Landscape
The interplay between ab, cd, and ef is a microcosm of the vast and beautiful world of geometry. It exemplifies the fundamental concepts of intersection, parallelism, and perpendicularity, which serve as building blocks for more complex geometric constructs. As you delve deeper into the fascinating world of geometry, you will discover a wealth of mathematical beauty and practical applications.
Are you ready to embark on an exciting journey of geometric exploration? Dive into the enigmatic world of straight lines and witness their captivating interactions firsthand.
