Quadratic equations, with their charmingly poised curves and intricate variables, are often perceived as mere abstract entities confined to the pages of algebra textbooks. However, beneath their mathematical guise, these equations hold a profound significance, orchestrating a symphony of real-world phenomena that shape our lives. From the graceful arc of a projectile to the optimal design of a satellite’s trajectory, quadratic equations dance behind the scenes, shaping our understanding of the physical world with remarkable precision.

Image: youvegotthismath.com
In the realm of physics, projectile motion stands as a captivating testament to the power of quadratic equations. As an object soars through the air, its parabolic path, elegantly described by the equation h = -1/2gt2 + vt + h0, embodies the principles of gravity and velocity. This understanding empowers us to predict the trajectory of everything from a soaring bird to a launched rocket, enabling us to navigate the world with accuracy and precision.
Engineering the Ideal Satellite Trajectory: A Quadratic Conundrum
The harmonious dance of quadratic equations weaves its intricate patterns into the world of engineering. When designing the path of a satellite, engineers meticulously employ these equations to optimize its trajectory. By calculating the satellite’s initial velocity and launch angle, they craft a parabolic path that gracefully delivers the satellite to its intended orbital position. This delicate balancing act ensures efficient use of fuel, maximizes the satellite’s lifespan, and unlocks the full potential of space exploration.
The Architectural Symphony of Parabolic Arches
Beyond their scientific prowess, quadratic equations find harmonious expression in architecture, an artistic realm where form meets mathematical elegance. From the majestic sweep of the Gateway Arch in St. Louis to the graceful curves of the Sydney Harbour Bridge, parabolic arches stand as testaments to the power of these equations to create breathtaking structures. By distributing weight evenly across the arch’s span, these equations enable architects to design bridges, domes, and vaulted ceilings that soar to impressive heights, creating iconic landmarks that inspire awe and wonder.
Unveiling the Secrets of Projectile Motion: A Parabolic Journey
The study of projectile motion offers a fascinating insight into the practical applications of quadratic equations. From humble beginnings with rocks skipping across water to the awe-inspiring launch of a spacecraft, the parabolic trajectory of projectiles is governed by the equation d = v0t – 1/2gt2, a testament to the ubiquitous presence of quadratics in the physical world. Predicting the trajectory of a thrown baseball or designing the launch of an interplanetary mission both hinge on mastering the principles of these equations.
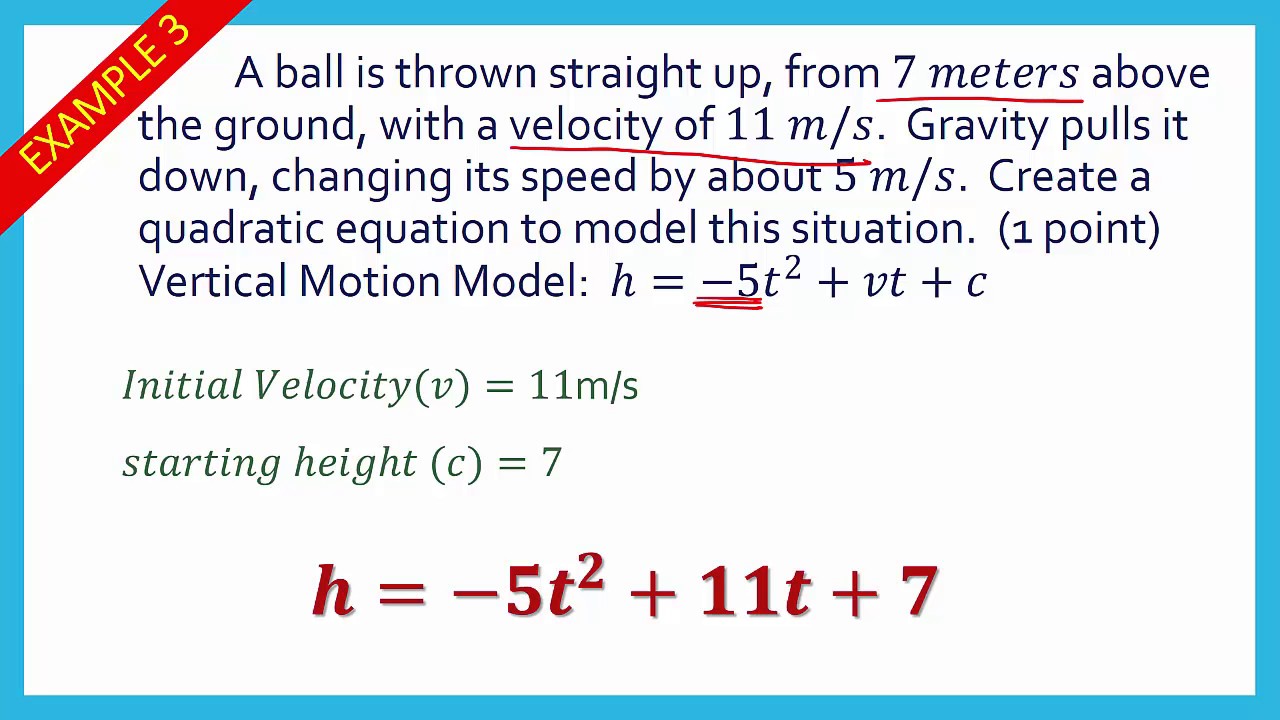
Image: www.slidemake.com
Hydrodynamics and the Art of Water Flow
As water flows through pipes and channels, its behavior is meticulously described by quadratic equations, a partnership that enables engineers to design efficient water distribution systems. By calculating the flow rate and pressure within pipes of varying diameters, these equations ensure an uninterrupted and dependable supply of water, a critical lifeline for modern society. Venturing into the world of river dynamics, quadratic equations elegantly model the flow and depth of rivers, aiding flood control measures and facilitating optimal dam design.
The Harmony of Electrical Circuits: A Quadratic Resonance
In the realm of electricity, quadratic equations hold the key to understanding the intricate dance of circuits. When an inductor and a capacitor are connected in a parallel circuit, the relationship between voltage and current is beautifully captured by the equation V = I(R + 1/ωC – ωL), a harmonious blend of quadratic principles and the fundamentals of alternating current. By manipulating these equations, engineers can design circuits with specific resonant frequencies, enabling the construction of filters, oscillators, and other electronic devices that play a crucial role in modern technology.
Landscaping Design: Sculpting Landscapes with Quadratic Precision
From manicured lawns to sculpted gardens, quadratic equations find unexpected application in landscaping design. By employing these equations, landscapers can meticulously craft hills and valleys, ensuring proper drainage and creating visually appealing outdoor spaces. Working hand in hand with nature, they transform raw terrain into breathtaking landscapes that offer a tranquil escape or serve as a stage for outdoor gatherings, bringing the precision of mathematics into the realm of aesthetics.
Demystifying Sound and the Secrets of Parabolas
Venturing into the realm of acoustics, quadratic equations provide the key to understanding the behavior of sound waves. When sound waves reflect off a parabolic surface, such as a satellite dish or a microphone, the resulting reflection follows a parabolic path, enabling precise focusing and directionality. This principle finds practical application in technologies such as radar, sonar, and medical imaging, allowing us to probe the depths of the ocean, detect distant objects, and visualize the intricate workings of the human body.
Finance and the Art of Quadratic Modeling
In the intricate world of finance, quadratic equations step into the spotlight as powerful tools for modeling complex relationships. From analyzing stock market trends to forecasting economic growth, these equations enable financial analysts to unravel patterns and make informed predictions. By incorporating quadratic terms into their models, they can capture the curvature of data points, leading to more accurate forecasts and potentially lucrative investment decisions.
Cybersecurity: Defending Digital Realms with Quadratic Safeguards
In the digital realm, quadratic equations play a crucial role in safeguarding data and protecting against cyberattacks. Encryption algorithms, the gatekeepers of secure communication, often employ quadratic principles to generate encryption keys that are virtually impossible to crack. These keys are the backbone of online banking, e-commerce, and countless other activities that rely on the secure transmission of sensitive information
Real Life Example Of A Quadratic Equation
Conclusion
As we delve into the captivating world of quadratic equations, their enigmatic powers unfold, revealing a universe beyond the confines of abstract mathematics. From the soaring trajectory of a soccer ball to the intricate design of a suspension bridge, these equations orchestrate a symphony of real-world phenomena. They empower us to unravel the mysteries of physics, inspire architectural marvels, optimize engineering designs, and safeguard our digital world. Embracing the harmonious dance of quadratic equations, we gain not only a deeper understanding of the natural world but also the power to shape it, forging a path towards countless innovations that will continue to redefine our collective future.
